How Tdo You Know When to Use Aq
Deviation Betwixt K And Q
- Page ID
- 1378
Sometimes it is necessary to make up one's mind in which direction a reaction will progress based on initial activities or concentrations. In these situations, the relationship between the reaction quotient, \(Q_c\), and the equilibrium constant, \(K_c\), is essential in solving for the net alter. With this human relationship, the direction in which a reaction will shift to attain chemical equilibrium, whether to the left or the right, tin be easily calculated.
Introduction
\(K_c\) can exist used calculate the terminal concentrations at equilibrium for a reaction using an Ice table and the natural progression of the reaction, from left to right or from correct to left. However, what if you exercise not know which style the reaction will progress? A elementary relationship between \(K_c\) and the reaction caliber, known as \(Q_c\), can help. The reaction quotient, \(Q\), expresses the relative ratio of products to reactants at a given instant. Using either the initial concentrations or initial activities of all the components of the reaction, the progression of an reaction can easily exist determined.
Given the full general chemical reaction
\[ aA +bB \rightleftharpoons gG +hH \]
\(Q\) may be expressed as the following equations:
\[ Q= \dfrac{a_{init}^ga_{init}^h}{a_{init}^a a_{init}^b} \]
or
\[ Q_{c}= \dfrac{[G]_{init}^g[H]_{init}^h}{[A]_{init}^a[B]_{init}^b} \]
Remember that the concentrations of liquids and solids do not modify, so they are excluded from the expression. As shown above, the value of \(Q\) can be found by raising the products to the ability of their coefficients, or stoichiometric factors, divided past the reactants raised to their coefficients. If the concentration of products in the numerator is much larger than that of reactants in the denominator, \(Q\) will be a big value. On the other hand, a small-scale amount of products (minor numerator) divided by a big value for the concentration of reactants (large denominator) would result in a small value for Q. The expressions for Q are very like to those for \(K\):
\[ K= \dfrac{a_G^g a_{H}^h}{a_{A}^a a_{B}^b} \]
or
\[ K_c = \dfrac{[G]^g [H]^h}{[A]^a [B]^b} \]
To determine which management a reaction volition go towards, only compare \(Q_c\), the initial concentration ratio, to \(K_c\), the equilibrium constant, and evaluate the results.
Q vs. K: What Does It Mean?
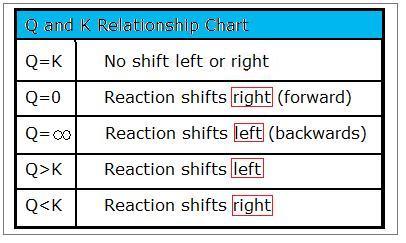
When you gear up \(Q\) against \(K\), there are five possible relationships:
- \(Q=K\)
- \(Q=0\)
- \(Q<K\)
- \(Q= \infty\) and
- \(Q>K\).
To properly predict which way a reaction will progress, you must know these relationships.
Situation ane: Q = M
When Q=K, the organization is at equilibrium and at that place is no shift to either the left or the right.
Take, for example, the reversible reaction shown beneath:
\[ CO_{(1000)}+2H_{2 \; (g)} \rightleftharpoons CH_{three}OH_{(chiliad)} \]
The value of Kc at 483 Grand is 14.5. If Q=xiv.5, the reaction is in equilibrium and will exist no evolution of the reaction either frontward or backwards.
State of affairs two: Q < K
When Q<Chiliad, there are more reactants than products. As a result, some of the reactants will become products, causing the reaction to shift to the right.
Consider again:
\[ CO_{(chiliad)}+2H_{2 \; (thou)} \rightleftharpoons CH_{iii}OH_{(g)} \]
For Q<1000:
\[ CO_{(g)}+2H_{2 \; (yard)} \longrightarrow CH_{3}OH_{(thou)} \]
so that equilibrium may be established.
Q Equals Cipher
If Q=0, then Q is less than K. Therefore, when Q=0, the reaction shifts to the right (forrad). An piece of cake fashion to remember this human relationship is by thinking, "once you accept nada, the just thing left to do is to move frontwards." If Q equals to zero, the reaction will shift forrard (to the right):
\[ CO_{(g)}+2H_{two \; (grand)} \longrightarrow CH_{three}OH_{(chiliad)} \]
Situation 3: Q > K
When Q>K, at that place are more products than reactants. To decrease the corporeality of products, the reaction will shift to the left and produce more reactants. For Q>K:
\[ CO_{(thou)}+2H_{2 \; (g)} \longleftarrow CH_{3}OH_{(g)} \]
Q Equals Infinity
When Q=∞, the reaction shifts to the left (backwards). This is a variation of when Q>>>K.
\[ CO_{(grand)}+2H_{2 \; (g)} \longleftarrow CH_{3}OH_{(g)} \]
Remembering the Human relationship Between K and Q
An piece of cake fashion to remember these relationships is by thinking of the > or < as the mouth of an alligator. The alligator volition "consume" in the direction that the reaction shifts as long as \(Q\) is written before \(K\).

Handy Nautical chart Outlining the Relationships of Q and Chiliad
Remembering these unproblematic relationships will assistance you to solving for the progression of a reaction. A chart outlining them can be found below.
Predicting the Shift of a Reaction Without Calculations
Depending on what a problem asks of you, sometimes information technology is unnecessary to brand any calculations at all. Accept, for example, the at present familiar reversible reaction listed below:
\[ CO_{(g)}+2H_{ii \; (grand)} \rightleftharpoons CH_{3}OH_{(g)} \]
What do you call back will happen if more of the product, methanol (CH3OH), is added? Equilibrium will be disrupted, and the increase in products mean that Q>K. In order to re-found equilibrium, the reaction volition progress to the left, towards the reactants. This ways some of the added methanol volition break downwards into carbon monoxide and hydrogen gas.
\[ CO_{(grand)}+2H_{2 \; (g)} \longleftarrow CH_{iii}OH_{(g)} \]
Now, what if more than of the reactants, carbon monoxide and hydrogen gas, are a? Yous should realize that this would upset the equilibrium. Q<K, considering the value for the amount of reactants, or the denominator of the Q expression, has increased. To plant equilibrium once more, the reaction will favor the product, so the reaction volition progress to the right.
\[ CO_{(g)}+2H_{2 \; (one thousand)} \longrightarrow CH_{3}OH_{(1000)} \]
The ideas illustrated above show Le Chatelier's Principle whereby when an equilibrated organisation is subjected to a change in temperature, pressure level, or concentration of a species in the reaction, the arrangement responds by achieving a new equilibrium that partially offsets the bear on of the modify. Predicting which way a reaction will go can exist the easiest thing that you lot will ever do in chemical science!
Example: Putting It All Together
To properly employ the human relationship between Q and Thousand, you must know how to fix it upwards. Have, for example, the reaction below:
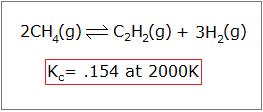
If y'all start with 4.00M CH4, 2.00M C2H2, and 3.00M H2, which direction will the reaction progress to achieve equilibrium?
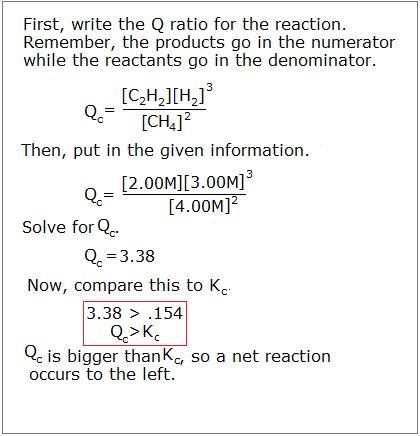
Problems
1) Consider this reaction:
\(2NOBr_{(g)} \leftrightharpoons 2NO_{(thou)}+Br_{2}\)
If Thouc= 0.0142 and the initial concentrations are 1.0 M NOBr, 0.2M NO, and 0.8M Br2, which way will the reaction progress to reach equilibrium?
2) What is Q and its purpose?
iii) Consider the reaction following in equilibrium:
\(N_{2}O_{4 \; (g)} \leftrightharpoons 2NO_{two \; (g)}\)
If more North2O4 is added, which way will the reaction proceed?
iv) Consider the following reaction:
\(CO_{(g)}+Cl_{2 \; (chiliad)} \leftrightharpoons COCl_{two \; (yard)}\)
With a Kc of 1.ii x x3 at 668 K, is the reaction in equilibrium when there are 5.00 mol CO(g), 2.00 mol Cl2(1000), and six.00 mol of COCl2(1000) in a 3.00L flask? If non, which direction will the reaction progress to achieve equilibrium?
5) Consider the following reaction:
\(H_{2 \; (chiliad)}+I_{two \; (g)} \leftrightharpoons 2HI_{(thousand)}\)
If Kc=50.two at 718 Thou and the initial concentrations are 0.v M Htwo, 0.15M Itwo, and 0.05M HI, which way will the reaction progress?
6) Consider the post-obit reaction:
\(2COF_{two \; (chiliad)} \leftrightharpoons CO_{2 \; (k)}+CF_{4 \; (g)}\)
If Kc= 2.00 at 473 Chiliad and the initial concentrations are 2.0 M COtwo, four.0 M CF4, and 0.5 1000 COF2, which way will the reaction progress?
7) Consider the following reaction:
\(2SO_{two \; (g)}+O_{ii \: (g)} \leftrightharpoons 2SO_{iii \; (1000)}\)
Kc=100. With the initial masses of xx g And then2, 13 yard O2, and 25 g So3 in a 5.0 L container, which way will the reaction progress.
Solutions
1) The reactions shifts to the left, towards the reactants.
NOBr= 1M, NO= 0.2M, Br2= 0.8M
\(Q_c= \dfrac {[0.2]^2 [0.8]}{[one]^2}\)
\(Q_c= 0.032\)
Therefore, Qc> Chiliadc and the reactions shifts towards the reactants.
two) Q is a reaction quotient, which helps make up one's mind if a reaction will shift forwards or backwards. Equally a organisation approaches towards equilibrium, Q approaches towards K.
three) The reaction will proceed to the right.
4) No, it is not at equilibrium. Since Q<K, the reaction volition shift to the correct to reach equilibrium.
5) Q = 0.033, and then Q<Chiliad. The reaction will shift to the correct.
six) Q = 32.0, so Q>K. The reaction will shift to the left.
vii) Q = 12, and then Q<K. The reaction volition shift to the right.
References
- Alberty, R., A. Cornish-Bowden, et al. (1994). "Recommendations for nomenclature and tables in biochemical thermodynamics." Pure Appl. Chem 66: 1641–1666.
- Gold, J. and V. Gold (1985). "Le Chatelier'southward Principle and the Laws of van't Hoff." Education in Chemistry 22: 82-85.
- Petrucci, Harwood, Herring, Madura. General Chemistry: Principles & MOdern Application. Ninth Edition. Pages 636-638.
Contributors and Attributions
- Rubi Medrano (UCD), Irene Ly (UCD)
Source: https://chem.libretexts.org/Bookshelves/Physical_and_Theoretical_Chemistry_Textbook_Maps/Supplemental_Modules_(Physical_and_Theoretical_Chemistry)/Equilibria/Chemical_Equilibria/Difference_Between_K_And_Q
0 Response to "How Tdo You Know When to Use Aq"
Post a Comment